Step-by-step explanation:
Let the first wave is :
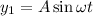
And another wave is :
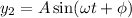
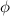 is phase difference between waves
is phase difference between waves
Let y is the resultant of these two waves. So,

The waves reflected from the upper and lower surfaces of the medium, it means that the resultant to be zero. So,

So, the phase difference between the two waves is
 .
.