Answer:
Option (3)
Explanation:
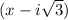 is a factor of a polynomial given in the options, that means a polynomial having factor as
is a factor of a polynomial given in the options, that means a polynomial having factor as
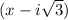 will be 0 for the value of x =
will be 0 for the value of x =
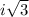 .
.
Option (1),
3x⁴ + 26x² - 9
=
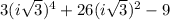 [For x =
[For x =
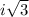 ]
]
= 3(9i⁴) + 26(3i²) - 9
= 27 - 78 - 9 [Since i² = -1]
= -60
Option (2),
4x⁴- 11x² + 3
=
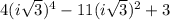
= 4(9i⁴) - 33i² + 3
= 36 + 33 + 3
= 72
Option (3),
4x⁴ + 11x² - 3
=

= 4(9i⁴) + 33i² - 3
= 36 - 33 - 3
= 0
Option (4),
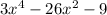
=
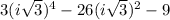
= 3(9i⁴) - 26(3i²) - 9
= 27 + 78 - 9
= 96
Therefore,
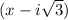 is a factor of option (3).
is a factor of option (3).