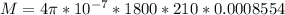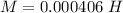Answer:
The mutual inductance is
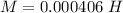
Step-by-step explanation:
From the question we are told that
The number of turns per unit length is
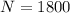
The radius is
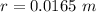
The number of turns of the solenoid is

Generally the mutual inductance of the system is mathematically represented as
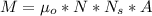
Where A is the cross-sectional area of the system which is mathematically represented as
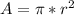
substituting values
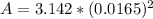

also
 is the permeability of free space with the value
is the permeability of free space with the value
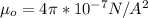
So