Answer:
3.54%
Explanation:
This question represents a binomial distribution. A binomial distribution is given by:
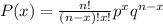
Where n is the total number of trials, p is the probability of success, q is the probability of failure and x is the number of success.
Given that:
A basketball player scores 70% of his shots on average, therefore p = 70% = 0.7. Also q = 1 - p = 1 - 0.7 = 0.3.
The total number of trials (n) = 20 shots
The probability that he scores at least 18 successful shots tonight if he gets 20 shots = P(x = 18) + P(x = 19) + P(x = 20)
P(x = 18) =

P(x = 19) =
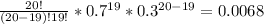
P(x = 20) =
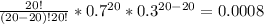
The probability that he scores at least 18 successful shots tonight if he gets 20 shots = P(x = 18) + P(x = 19) + P(x = 20) = 0.0278 + 0.0068 + 0.0008 = 0.0354 = 3.54%