Answer:
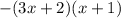
Explanation:
So we have the polynomial:
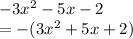
(I moved the negative to the outside. This is optional, but it makes things cleaner and you will have to do it eventually.)
This is a quadratic. To factor this, we need to find two numbers p and q such that:

From there, we can substitute these numbers in for b.
From the quadratic, a=3, b=5, and c=2. We ignore the negative sign.
In other words, ac=6 and b=5.
After guessing and checking, two numbers that work are 3 and 2 since 3(2)=6 ad 3+2=5. We substitute these values in for b. So:
