Question

Use the ratio of a 30-60-90 triangle to solve for the variables. Leave your answers as radicals in simplest form. x= y=

Answer 1

x = 10 units, y = 5 units

Explanation:

Given triangle ABC is a 30-60-90 triangle,

m∠C = 60°

By applying Sine rule in the given triangle,

Sin(60)°=
`\frac{\text{Opposite side}}{\text{Hypotenuse}}`

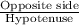
Sin(60)°
`=\frac{\text{AB}}{\text{AC}}`

`(√(3))/(2)=\frac{\text{AB}}{\text{AC}}`

`(√(3))/(2)=(5√(3))/(x)`

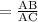
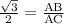
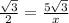
x = 10 units

Similarly, by applying Cosine rule in the given triangle,

Cos(60)° =
`\frac{\text{Adjacent side}}{\text{Hypotenuse}}`

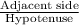
Cos(60)° =
`=\frac{\text{BC}}{\text{AC}}`

`(1)/(2)=(y)/(x)`

y =
`(x)/(2)`

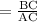
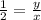
y = 5 units

Therefore, x = 10 units and y = 5 units will be the answer.