Answer: 0.0548
Explanation:
Given, A research study investigated differences between male and female students. Based on the study results, we can assume the population mean and standard deviation for the GPA of male students are µ = 3.5 and σ = 0.05.
Let
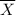 represents the sample mean GPA for each student.
represents the sample mean GPA for each student.
Then, the probability that the random sample of 100 male students has a mean GPA greater than 3.42:
![P(\overline{X}>3.42)=P(\frac{\overline{X}-\mu}{(\sigma)/(√(n))}>(3.42-3.5)/((0.5)/(√(100))))\\\\=P(Z>(-0.08)/((0.5)/(10)))\ \ \ [Z=\frac{\overline{X}-\mu}{(\sigma)/(√(n))}]\\\\=P(Z>1.6)\\\\=1-P(Z<1.6)\\\\=1-0.9452=0.0548](https://img.qammunity.org/2021/formulas/mathematics/college/j9ylqhkblce832184uu8hf6lqz6w3u6sve.png)
hence, the required probability is 0.0548.