Question

Find the equation of the line: With an x intercept of 4 and ay intercept of −1.5.

Answer 1

y = (3/8)x - 1.5

Explanation:

Use the slope-intercept form y = mx + b. Substitute -1.5 for b, 4 for x and 0 for y. Then: 0 = m(4) - 1.5, or

4m = 1.5, or

The slope is m = 1.5/4, or m = 0.375, or m = 3/8.

Note that the x-intercept can be treated just like any other point: (4, 0)

Then the desired equation is y = (3/8)x - 1.5

Answer 2

`\boxed{y=(3)/(8) x-1.5}`

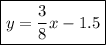
Explanation:

Part 1: Determining slope from two given points

We are given the points
`x=4` and
`y=-1.5`. We can go ahead and make the first part of the equation because we are given one of the unknowns (the y-intercept, or
`b`). The equation becomes
`y=mx-1.5`.

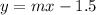Part 2: Determine the coordinate points

`x=4` is a x-intercept, meaning it crosses the x-axis at this point. The y-value is
`0` ⇒
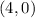`(4, 0)` is the first point.

`y=-1.5` is a y-intercept, meaning it crosses the y-axis at this point. The x-value is
`0` ⇒
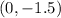`(0, -1.5)` is the second point.

Now, plug these values into the point-slope formula:
`m=(y_(2) -y_(1) )/(x_(2)-x_(1))`

`m=(-1.5 - 0)/(0 - 4)`

`m = (-1.5)/(-4)`

`m = (3)/(8)`

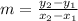
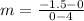
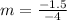
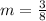
Plug this information into the equation to get your final answer of
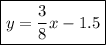`\boxed{y=(3)/(8) x-1.5}`.