Question

three people are watching a hot air balloon travel over their town. at a certain point in time, one person stands directly below the balloon, and the others look at it at certain angles. in the following image, a,b, and c are people, and d is the balloon. person c is 384m directly below the balloon, person b is 200m away from person c, and the angle between person a, the balloon, and person b is 33 degrees. how far is person a from the hot air balloon

Answer 1

Distance between balloon and a is = 383.67 m

Explanation:

The given situation can be represented as the given diagram as attached in the answer area.

cd = 384 m

cb = 200 m

`\angle adb = 33^\circ`

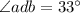
To find:

Distance between balloon and a i.e. side ad = ?

Solution:

First of all, let us consider the right angled
`\triangle bcd`.

We know the trigonometric identity that:

`tan\theta = (Perpendicular)/(Base)`

`tan\angle cbd =(cd)/(cb)\\\Rightarrowtan\angle cbd =(384)/(200)\\\Rightarrowtan\angle cbd =1.92\\\Rightarrow \angle cbd = tan^(-1)(1.92) = 62.49^\circ`

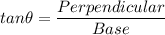
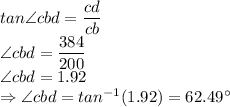
Now, using the external angle property for the external
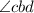`\angle cbd` for the
`\triangle abd`:

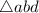(External angle is equal to the sum of two opposite angles of the triangle.)

`\angle cbd = \angle adb+\angle a`

`\Rightarow \angle a =62.49-33 =29.49^\circ`

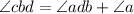
Now, let us consider the right angled
`\triangle acd`.

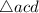We have the value of
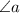`\angle a` and perpendicular dc.

We have to find the hypotenuse ad.

Let us use the sine identity:

`sin\theta =(Perpendicular)/(Hypotenuse)\\\Rightarrow sin\angle a =(cd)/(ad)\\\Rightarrow sin(29.49^\circ) =(384)/(ad)\\\Rightarrow ad = (384)/(0.49)\\\Rightarrow \bold{ad = 783.67\ m}`

So, the answer is:

Distance between balloon and
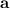`\bold{a}` is = 383.67 m