Answer:
a) 2.278 x 10^-5 volts
b) 1.139 x 10^-6 Ampere
c) 2.59 x 10^-11 W
Step-by-step explanation:
The radius of the wire r = 2 mm = 0.002 m
the number of turns N = 200 turns
direction of the magnetic field ∅ = 25°
magnetic field strength B = 0.02 T
varying time = 2 sec
The cross sectional area of the wire =

==> A = 3.142 x
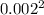 = 1.257 x 10^-5 m^2
= 1.257 x 10^-5 m^2
Field flux Φ = BA cos ∅ = 0.02 x 1.257 x 10^-5 x cos 25°
==> Φ = 2.278 x 10^-7 Wb
The induced EMF is given as
E = NdΦ/dt
where dΦ/dt = (2.278 x 10^-7)/2 = 1.139 x 10^-7
E = 200 x 1.139 x 10^-7 = 2.278 x 10^-5 volts
b) If the two ends are connected to a resistor of 20 Ω, the current through the resistor is given as
 = E/R
= E/R
where R is the resistor
 = (2.278 x 10^-5)/20 = 1.139 x 10^-6 Ampere
= (2.278 x 10^-5)/20 = 1.139 x 10^-6 Ampere
c) power delivered to the resistor is given as
P =
 E
E
P = (1.139 x 10^-6) x (2.278 x 10^-5) = 2.59 x 10^-11 W