Question

A researcher is interested in determining the mean energy consumption of a new

compact florescent light bulb. She takes a random sample of 41 bulbs and determines
that the mean consumption is 1.3 watts per hour with a standard deviation of 0.7.
When constructing a 97% confidence interval, which would be the most appropriate
value of the critical value?

A) 1.936
B) 2.072
C) 2.250
D) 2.704
E) 2.807

Answer 1

The most appropriate value of the critical value is 2.289.

Explanation:

We are given that a researcher takes a random sample of 41 bulbs and determines that the mean consumption is 1.3 watts per hour with a standard deviation of 0.7.

We have to find that when constructing a 97% confidence interval, which would be the most appropriate value of the critical value.

Firstly, as we know that the test statistics that would be used here is t-test statistics because we don't know about the population standard deviation.

So, for finding the critical value we will look for t table at (41 - 1 = 40) degrees of freedom at the level of significance will be
`(1 - 0.97)/(2) = 0.015` .

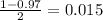Now, as we can see that in the t table the critical values for P = 1.5% are not given, so we will interpolate between P = 2.5% and P = 1%, i.e;

`(0.015 - 0.025)/(0.025-0.01)= \frac{\text{Critcal value}-2.021}{2.021-2.423}`

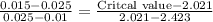
So, the critical value at a 1.5% significance level is 2.289.