Answer:
The answer is below
Explanation:
Given that mean (μ) = 266 days, standard deviation (σ) = 16 days, sample size (n) = 16 women.
a) The mean of the sampling distribution of Xbar (
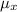 ) is given as:
) is given as:
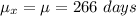
The standard deviation of the sampling distribution of Xbar (
 ) is given as:
) is given as:
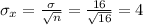
b) The z score is a measure in statistics used to determine by how much the raw score is above or below the mean. It is given by:
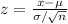
For x > 270 days:
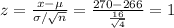
The probability the average pregnancy length exceed 270 days = P(x > 270) = P(z > 1) = 1 - P(z < 1) = 1 - 0.8413 = 0.1587 = 15.87%