Question

a political candidate has asked you to conduct a poll to determine what percentage of people support her. if the candidate only wants a 8% margin of error at a 95% cnofidence level, what size of sample is needed

Answer 1

The sample needed is
`n =150`

Explanation:

From the question we are told that

The margin of error is
`E = 0.08`

The confidence level is
`C = 95 \% = 0.95`

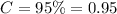
Given that the confidence level is 95% the level of significance is mathematically represented as

`\alpha = 1 - 0.95`

`\alpha = 0.05`

Next we obtain the critical value of
`(\alpha )/(2)` from the z-table , the value is
`Z_{(\alpha )/(2) } = 1.96`

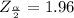
The reason we are obtaining critical value of
`(\alpha )/(2)` instead of
`\alpha` is because

`\alpha` represents the area under the normal curve where the confidence level interval (
`1-\alpha` ) did not cover which include both the left and right tail while

`(\alpha )/(2)` is just the area of one tail which what we required to calculate the margin of error

The sample size is mathematically represented as

`n = [\frac{Z_{(\alpha )/(2) }}{E} ]^2 * \r p[1-\r p]`

Here
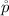`\r p` is sample proportion of people that supported her and we will assume this to be 50% = 0.5

So

`n = [(1.96)/( 0.08) ]^2 * [0.5 (1- 0.5)]`

`n =150`