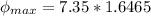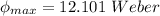Question

A uniform magnetic field of 7.35 T points in some direction. Consider the magnetic flux through a large triangular wire loop that has three equal sides of 1.95 m. Determine the maximum of the absolute value of the magnetic flux.

Answer 1

The maximum of the absolute value of the magnetic flux is
`\phi_(max) = 12.10 1 \ Weber`

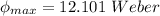
Step-by-step explanation:

From the question we are told that

The magnetic field is
`B = 7.35 \ T`

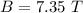
One side of the triangle is
`d = 1.95 \ m`

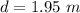
Generally the absolute value of the magnetic flux is mathematically represented as

`\phi = B * A cos (\theta _1 )`

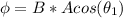
At maximum
`\theta_1 = 0`

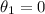
So

The maximum of the absolute value of the magnetic flux.

`\phi_(max) = B * A`

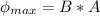
Now we are told that the triangle is of equal sides so the angle each makes with the other is
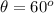`\theta = 60 ^o` this is because total angle in a triangle is 180

Now the height of the triangular loop is mathematically evaluated using SOHCAHTOA as

`sin \theta =(h)/(d)`

=>
`sin( 60)=(h)/(1.95)`

=>
`h = sin (60) * 1.95`

=>
`h = 1.6887 \ m`

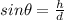
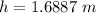
Hence the area is evaluated as

`A = (1)/(2) * d * h`

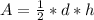
substituting values

`A = 0.5 * 1.95 * 1.6887`

`A = 1.6465 \ m^2`

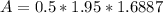
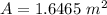
Thus

`\phi_(max) = 7.35 * 1.6465`

`\phi_(max) = 12.10 1 \ Weber`