Answer:
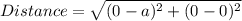
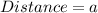
Explanation:
Given
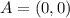


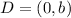
Required
Determine the distance between A and B
The distance between points is calculated as thus;
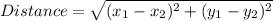
Considering points A and B
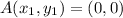
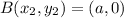
Substitute values for
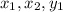 and
and
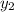 in the above formula
in the above formula
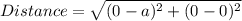
The above expression represents the distance between A and B;
However, it can be solved further as thus
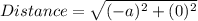
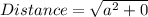
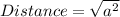
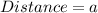
Hence, the distance between A and B is a