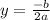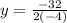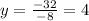Answer:

Explanation:
Axis of symmetry is a line that cuts the parabola in half touching the vertex.
Quadratic forms ⇒ y = ax² + bx + c or x = ay² + by + c
Axis of symmetry ⇒ x =
 or y =
or y =

First problem:
y = -3(x+3)²-2
Write in quadratic form ⇒ y = ax² + bx + c
y = -3(x² + 6x + 9) - 2
y = -3x² -18x - 27 - 2
y = -3x² -18x - 29
a = -3, b = -18
Find axis of symmetry.
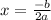

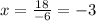
Second problem:
x = -4(y -4)² +6
Write in quadratic form ⇒ x = ay² + by + c
x = -4(y² - 18y + 16) + 6
x = -4y² + 32y - 64 + 6
x = -4y² + 32y - 58
a = -4, b = 32
Find axis of symmetry.