Answer: The concentration will be 8 milligrams per liter at 2 hours and 4 hours.
Explanation:
GIven: The concentration of a certain painkiller supplied by serum varies in its effectiveness over time according to the following function,
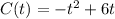 where C is the concentration of the painkiller in the serum measured in milligrams per liter so that it takes effect during t hours.
where C is the concentration of the painkiller in the serum measured in milligrams per liter so that it takes effect during t hours.
Put C(t)=8, then we get
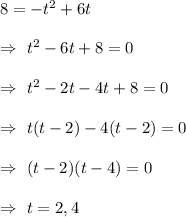
At t=2,
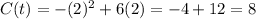
At t=4,
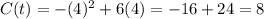
Hence, the concentration will be 8 milligrams per liter at 2 hours and 4 hours.