Question

A skateboarder jumps horizontally off the top of a staircase and lands at bottom of the stairs. The staircase has a horizontal length of 12.0 m, and the jump lasts 1.10s. We can ignore air resistance. What is the skater's vertical velocity upon landing? Round your answer to three significant digits.

Answer 1

-10.8

Step-by-step explanation:

Answer on Khan Academy

Answer 2

The vertical velocity of the skater upon landing is 10.788 meters per second.

Step-by-step explanation:

Skateboarder experiments a parabolic movement. As skateboarder jumps horizontally off the top of the staircase, it means that vertical component of initial velocity is zero and accelerates by gravity, the final vertical speed is calculated by the following expression:

`v = v_(o) + g\cdot t`

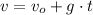
Where:

`v_(o)` - Initial vertical speed, measured in meters per second.

`v` - Final vertical speed, measured in meters per second.

`g` - Gravitational acceleration, measured in meters per square second.

`t` - Time, measured in seconds.

Given that
`v_(o) = 0\,(m)/(s)`,
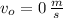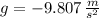`g = -9.807\,(m)/(s^(2))` and
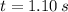`t = 1.10\,s`, the final velocity of the skater upon landing is:

`v = 0\,(m)/(s) + \left(-9.807\,(m)/(s^(2)) \right)\cdot (1.10\,s)`

`v = -10.788\,(m)/(s)`

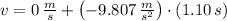
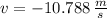
The vertical velocity of the skater upon landing is 10.788 meters per second.