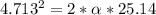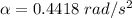Question

A record player rotates a record at 45 revolutions per minute. When the record player is switched off, it makes 4.0 complete turns at a constant angular acceleration before coming to rest. What was the magnitude of the angular acceleration (in rads/s2) of the record as it slowed down

Answer 1

The angular acceleration is
`\alpha = 0.4418 \ rad /s^2`

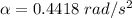
Step-by-step explanation:

From the question we are told that

The angular speed is
`w_f = 45 \ rev / minutes = (45 * 2 * \pi )/(60 )= 4.713 \ rad/s`

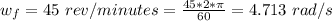
The angular displacement is
`\theta =4 \ rev = 4 * 2 * \pi = 25.14 \ rad`

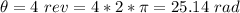
From the first equation of motion we can define the movement of the record as

`w_f ^2 = w_o ^2 + 2 * \alpha * \theta`

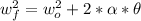
Given that the record started from rest
`w_o = 0`

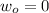
So

`4.713^2 = 2 * \alpha * 25.14`

`\alpha = 0.4418 \ rad /s^2`