Answer:

Explanation:
Hello, please find below my work.

We know that for any a and x real numbers we can write
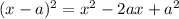
When we compare with the left part of the equation we can identify the term in x so that -4=-2a (multiply by -1) <=>4=2a (divide by 2) <=> a = 4/2 = 2
So we can write
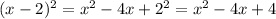
So we have to add 4 to both sides of the equation to complete the square and it comes:

Hope this helps.
Do not hesitate if you need further explanation.
Thank you