Question

A centrifugal pump is operating at a flow rate of 1 m3/s and a head of 20 m. If the specific weight of water is 9800 N/m3 and the pump efficiency is 85%, the power required by the pump is most nearly:

Answer 1

The power required by the pump is nearly 230.588 kW

Step-by-step explanation:

Flow rate of the pump Q = 1 m^3/s

the head flow H = 20 m

specific weight of water γ = 9800 N/m^3

efficiency of the pump η = 85%

First note that specific gravity of water is the product of the density of water and acceleration due to gravity.

γ = ρg

where ρ is density. For water its value is 1000 kg/m^3

g is the acceleration due to gravity = 9.81 m/s^2

The power to lift this water at this rate will be gotten from the equation

P = ρgQH

but ρg = γ

therefore,

P = γQH

imputing values, we'll have

P = 9800 x 1 x 20 = 196000 W

But the centrifugal pump that will be used will only be able to lift this amount of water after the efficiency factor has been considered. The power of pump needed must be greater than this power.

we can say that

196000 W is 85% of the power of the pump power needed, therefore

196000 = 85% of
`P_(p)`

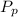
where
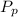`P_(p)` is the power of the pump needed

85% = 0.85

196000 = 0.85
`P_(p)`

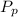
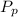`P_(p)` = 196000/0.85 = 230588.24 W

Pump power = 230.588 kW