Question

Construct a 99% confidence interval of the population proportion at the given level of confidence. x=240​, n=300 the lower bound is? the upper bound is?

Answer 1

Answer: lower bound = 0.7404; upper bound = 0.8596

Explanation:

The proportion p for this population:

p =
`(240)/(300)`

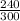
p = 0.8

Confidence interval for proportion is calculated as:

p ± z-score.
`\sqrt{(p(1-p))/(n) }`

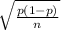
Z-score for a 99% confidence interval is: z = 2.58

Calculating:

0.8 ± 2.58.
`\sqrt{(0.8(0.2))/(300) }`

0.8 ± 2.58.
`√(0.00053)`

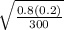
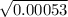
0.8 ± 2.58(0.0231)

0.8 ± 0.0596

This means that the lower limit of this interval is 0.7404 and upper bound is 0.8596