Question

Suppose that a 117.5 kg football player running at 6.5 m/s catches a 0.43 kg ball moving at a speed of 26.5 m/s with his feet off the ground, while both of them are moving horizontally.

(a) Calculate the final speed of the player, in meters per second, if the ball and player are initially moving in the same direction.
(b) Calculate the change in kinetic energy of the system, in joules, after the player catches the ball.
(c) Calculate the final speed of the player, in meters per second, if the ball and player are initially moving in opposite directions.
(d) Calculate the change in kinetic energy of the system, in joules, in this case.

Answer 1

a) 6.57 m/s

b) 53.75 J

c) 6.37 m/s

d) -98.297 J

Step-by-step explanation:

mass of player =
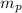`m_(p)` = 117.5 kg

speed of player =
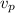`v_(p)` = 6.5 m/s

mass of ball =
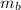`m_(b)` = 0.43 kg

velocity of ball =
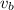`v_(b)` = 26.5 m/s

Recall that momentum of a body = mass x velocity = mv

initial momentum of the player = mv = 117.5 x 6.5 = 763.75 kg-m/s

initial momentum of the ball = mv = 0.43 x 26.5 = 11.395 kg-m/s

initial kinetic energy of the player =
`(1)/(2) mv^(2)` =
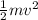`(1)/(2)` x 117.5 x
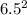`6.5^(2)` = 2482.187 J

a) according to conservation of momentum, the initial momentum of the system before collision must equate the final momentum of the system.

for this first case that they travel in the same direction, their momenta carry the same sign

`m_(p)`
`v_(p)` +
`m_(b)`
`v_(b)` = (
`m_(p)` +
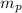
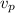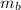
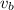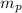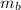`m_(b)`)v

where v is the final velocity of the player.

inserting calculated momenta of ball and player from above, we have

763.75 + 11.395 = (117.5 + 0.43)v

775.145 = 117.93v

v = 775.145/117.93 = 6.57 m/s

b) the player's new kinetic energy =
`(1)/(2) mv^(2)` =
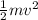`(1)/(2)` x 117.5 x
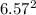`6.57^(2)` = 2535.94 J

change in kinetic energy = 2535.94 - 2482.187 = 53.75 J gained

c) if they travel in opposite direction, equation becomes

`m_(p)`
`v_(p)` -
`m_(b)`
`v_(b)` = (
`m_(p)` +
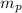
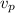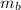
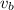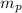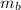`m_(b)`)v

763.75 - 11.395 = (117.5 + 0.43)v

752.355 = 117.93v

v = 752.355/117.93 = 6.37 m/s

d) the player's new kinetic energy =
`(1)/(2) mv^(2)` =
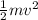`(1)/(2)` x 117.5 x
`6.37^(2)` = 2383.89 J

change in kinetic energy = 2383.89 - 2482.187 = -98.297 J

that is 98.297 J lost