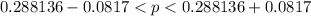Answer:
The confidence interval is

Explanation:
From the question we are told that
The sample is n = 118
The confidence level is C = 95 %
The number of people with high blood pressure is k = 34
The proportion of those with high blood pressure is evaluated as
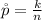
substituting values
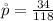
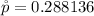
Given that the confidence level is 95% then the level of significance is evaluated as
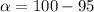
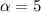 %
%

Now the critical values of
 obtained from the normal distribution table is
obtained from the normal distribution table is
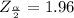
The reason we are obtaining values for is because is the area under the normal distribution curve for both the left and right tail where the 95% interval did not cover while is the area under the normal distribution curve for just one tail and we need the value for one tail in order to calculate the confidence interval
Now the margin of error is evaluated as

substituting values
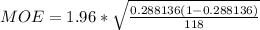
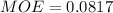
Thus the 95% confidence interval for the true percentage of all adults that have high blood pressure is evaluated as
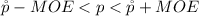
substituting values