Answer:
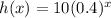
Explanation:
The exponential function h, represented in the table, can be written as

From table, at x=0, h(x) =10
Put theses values in equation,, we get
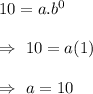
Also, for x= 1 , h(x) = 4, so put these values and a=10 in the equation , we get
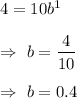
Put value of a and b in the equation ,
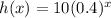 → Required equation.
→ Required equation.