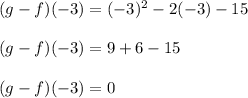Question

Find the difference of functions at x= - 3, (g - f)(-3), given f(x) and g(x): g(x) = x^2−15, and f(x) =2x

Answer 1

0

Explanation:

Solution:-

We are given two functions as follows:

`f ( x ) = x^2 - 15\\\\g ( x ) = 2x`

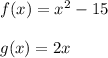
We need to determine the composite function defined as ( g - f ) ( x ). To determine this function we need to make sure that both function exist for all real positive value of x.

The function f ( x ) is a quadratic function which has real values for all values of x. Similarly, function g ( x ) is a linear line that starts from the origin. Hence, both functions are defined over the domain ( -∞, ∞ )

We will perform arithmetic operation of subtracting function f ( x ) from g ( x ) as follows:

`[ g - f ] ( x ) = g ( x ) - f ( x )\\\\\\( g - f ) ( x ) = x^2 - 15 - 2x\\\\`

Now evaluate the above determined function at x = -3 as follows:

`( g - f ) ( -3 ) = ( -3 )^2 - 2 ( -3 ) - 15\\\\( g - f ) ( -3 ) = 9 + 6 - 15\\\\( g - f ) ( -3 ) = 0`