Answer:
According to steps 2 and 4. The second-order polynomial must be added by
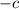 and
and
 to create a perfect square trinomial.
to create a perfect square trinomial.
Explanation:
Let consider a second-order polynomial of the form
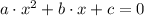 ,
,
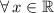 . The procedure is presented below:
. The procedure is presented below:
1)
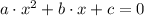 (Given)
(Given)
2)
 (Compatibility with addition/Existence of additive inverse/Modulative property)
(Compatibility with addition/Existence of additive inverse/Modulative property)
3)
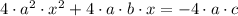 (Compatibility with multiplication)
(Compatibility with multiplication)
4)
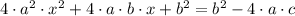 (Compatibility with addition/Existence of additive inverse/Modulative property)
(Compatibility with addition/Existence of additive inverse/Modulative property)
5)
 (Perfect square trinomial)
(Perfect square trinomial)
According to steps 2 and 4. The second-order polynomial must be added by
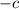 and
and
 to create a perfect square trinomial.
to create a perfect square trinomial.