Answer:
a) 0.35
b) 420 drawing pins
Explanation:
a) The question relates to the relative frequency of the pins pointing up which is found by the formula as follows;
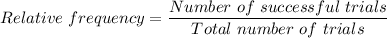
The total number of pins land 'point up' = 3 + 5 + 6 + 2 + 4 + 7 + 3 + 3 + 4 + 5
The total number of pins land 'point up' = 42 successful trials
Total number of trials = 12 × 10 = 120 trials

Due to the large number of trials, the relative frequency ≈ Probability and the probability that a single pin will land point up = 7/20 = 0.35
b) Given that the number of trials now = 12 × 100 = 1200, we have;
The expected number of drawing pins in total that would land point up = 0.35×1200 = 420 pins.