Question

The admission fee at an amusement park is $1.50 for children and S4 for adults. On a certain day, 289 people entered the park, and the admission fees collected totaled 746.00 dollars. How many children and

how many adults were admitted?
number of children equals
number of adults equals?

Answer 1

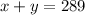`x+y = 289` (1) total people entered

`1.50 x +4 y = 746` (2) total amount collected

From the first equation we can solve for x and we got:

`x = 289-y` (3)

Replacing (3) into (2) we got:

`1.5(289-y) +4y = 746`

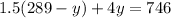
And solving for y we got:

`433.5 -1.5 y +4y = 746`

`2.5 y= 312.5`

`y=(312.5)/(2.5)= 125`

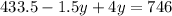
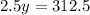
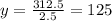
And then using (3) we can solve for x and we got:

`x= 289-125= 164`

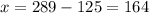
So then we have:

number of children = 164

number of adults = 125

Explanation:

Let x the number of children and y the number of adults. From the info given we can set up the following equations:

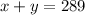`x+y = 289` (1) total people entered

`1.50 x +4 y = 746` (2) total amount collected

From the first equation we can solve for x and we got:

`x = 289-y` (3)

Replacing (3) into (2) we got:

`1.5(289-y) +4y = 746`

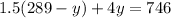
And solving for y we got:

`433.5 -1.5 y +4y = 746`

`2.5 y= 312.5`

`y=(312.5)/(2.5)= 125`

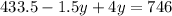
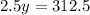
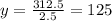
And then using (3) we can solve for x and we got:

`x= 289-125= 164`

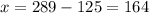
So then we have:

number of children = 164

number of adults = 125

Answer 2

Set up two equations:

Let a = adults and c = child:

a + c = 289 ( rewrite as a = 289 - c)

1.50c + 4a = 746

Replace a with the rewritten formula:

1.50c + 4(289-c) = 746

SImplify:

1.50c + 1156 - 4c = 746

Combine like terms:

-2.50c + 1156 = 746

Subtract 1156 from both sides:

-2.50c = -410

Divide both sides by -2.50

c = -410 / -2.50 = 164

Number of children = 164

Number of adults = 289 - 164 = 125