Answer:
John is 9, Brian is 6.
Explanation:
I)
Let
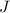 represent John's age and
represent John's age and
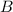 represent Brian's age.
represent Brian's age.
John is three years older than Brian. In other words:
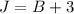
The product of their ages is 54. Or:

II)
Write this as a quadratic by substituting:
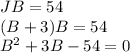
III)
Solve the quadratic:
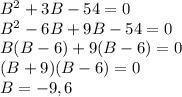
Since age cannot be negative, Brian must be 6 years old right now.
John is three year older, so John is 9.