Question

I REALLY NEED HELP! PLEASE help me...

Answer 1

A. The domain is (1,∞), and the range is (-7,∞)

Explanation:

Well lets graph it first,

Look at the image below ↓

By looking at the image we move it 3 units right and 3 units down.

Then it will be located at the point (1,-7).

Meaning for the domain it starts at 1 and goes on for infinity.

And For the range it starts down at -7 and goes down for infinity.

Thus,

the correct answer is choice A.

Hope this helps :)

Answer 2

A

Explanation:

Solution:-

- First we will go through the guidelines that are followed when a given function [ f ( x ) ] is translated in a cartesian coordinate system domain.

Horizontal shifts:

• Left shift: f ( x ) - > f ( x + a ).

• Right Shift: f ( x ) - > f ( x - a )

Where, the constant ( a ) denotes the magnitude of shift

Vertical shifts:

• Up shift: f ( x ) - > f ( x ) + b

• Down Shift: f ( x ) - > f ( x ) - b

Where, the constant ( b ) denotes the magnitude of shift

- The generalized form of a translated function is defined by the combination of both horizontal and vertical shifts as follows:

General: f ( x ) -> f ( x ± a ) ± b

Where, (a) and (b) are constants of respective translation shifts.

- We are given a function H ( x ) is to be translated 3 units to right and 3 units down. Use the above guidelines to determine the translated function H* ( x ) as follows:

`H ( x ) = √(x+2) - 4\\\\H^* ( x ) = H ( x - 3 ) - 3`

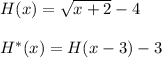
- Substitute ( x - 3 ) in place of all ( x ) in the given function H ( x ) and subtract ( 3 ) from H ( x ) as follows:

`H^* ( x ) = √(x-3 + 2) -4 - 3\\\\H^* ( x ) = √(x-1) -7\\`

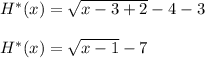
- Now we will look for any transcendental functions in the translated function H*(x). These are " Radicals, fractions, Logs, trigonometric ratios "

- We have a radical - > " square root " in H* ( x ). To find the domain of H*(x) we need to determine for what real values of x is the function H*(x) is defined.

- The square root exist for all only positive numbers. So the terms under the square root must be positive; hence,

`x - 1 \geq 0\\\\x \geq 1`

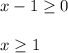
- Since the square root is the only transcendental in the given function H*(x) we have a one sided closed interval for the domain of the translated function.

Domain: [ 1 , ∞ ) ... Answer

- The range of the function is the corresponding output of function H*(x) for the domain established above. We can determine this by plugging in the end-points of the defined domain in the translated function H*(x) as follows:

`H^* ( 1 ) = √(1 - 1) - 7 = -7\\\\H^* ( inf ) = √(inf - 1) - 7 = inf - 7 = inf\\\\`

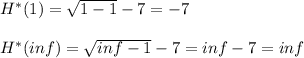
Therefore the range of the function is also a one sided closed interval bounded by x = 1.

Range: [-7 , ∞ ) ... Answer