Answer:
The correct substitution of a, b, and c in the quadratic formula is given by
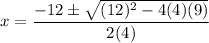
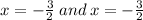
The solutions of the given quadratic equation are real and equal.
Explanation:
The given quadratic equation is
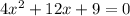
The coefficients a, b and c are as follow:
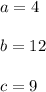
The quadratic formula is given by

The correct substitution of a, b, and c in the quadratic formula is given by
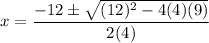
Bonus:
The solution of this quadratic equation is given by

Therefore, the solutions of the given quadratic equation are real and equal.