Question

Find the value of EB

Answer 1

31

Explanation:

Given,

AD = 38

EB = 7x - 4

FC = 6x - 6

Now, we have to find the value of X

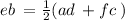`eb \: = (1)/(2) (ad \: + fc \: )` ( Mid segment Theorem )

Plug the values

`7x - 4 = (1)/(2) (38 + 6x - 6)`

Calculate the difference

`7x - 4 = (1)/(2) (32 + 6x)`

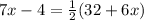
Remove the parentheses

`7x - 4 = (32)/(2) + (6x)/(2)`

`7x - 4 = 16 + 3x`

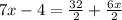
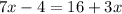
Move variable to L.H.S and change its sign

Similarly, Move constant to R.H.S and change its sign

`7x - 3x = 16 + 4`

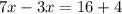
Collect like terms

`4x = 16 + 4`

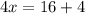
Calculate the sum

`4x = 20`

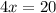
Divide both sides of the equation by 4

`(4x)/(4) = (20)/(4)`

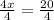
Calculate

`x = 5`

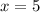
The value of X is 5

Now, let's find the value of EB

EB = 7x - 4

Plug the value of X

`= 7 * 5 - 4`

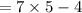
Calculate the product

`= 35 - 4`

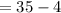
Calculate the difference

`= 31`

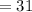
The value of EB is 31

Hope this helps..

Best regards!!