Question

. The client was hoping for a likability score of at least 5.2. Use your sample mean and standard deviation identified in the answer to question 1 to complete the following table for the margins of error and confidence intervals at different confidence levels. Note: No further calculations are needed for the sample mean. (6 points: 2 points for each completed row) Confidence Level | Margin of error | Center interval | upper interval | Lower interval 68 95 99.7

Answer 1

The 68% confidence interval is (6.3, 6.7).

The 95% confidence interval is (6.1, 6.9).

The 99.7% confidence interval is (5.9, 7.1).

Explanation:

The Central Limit Theorem states that if we have a population with mean μ and standard deviation σ and take appropriately huge random-samples (n ≥ 30) from the population with replacement, then the distribution of the sample-means will be approximately normally distributed.

Then, the mean of the sample means is given by,

`\mu_(\bar x)=\bar x`

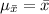
And the standard deviation of the sample means (also known as the standard error)is given by,

`\sigma_(\bar x)=(\sigma)/(√(n)) \ \text{or}\ (s)/(√(n))`

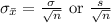
The information provided is:

`n=400\\\\\bar x=6.5\\\\s=4`

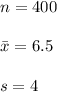
As n = 400 > 30, the sampling distribution of the sample-means will be approximately normally distributed.

(a)

Compute the 68% confidence interval for population mean as follows:

`CI=\bar x\pm z_(\alpha/2)\cdot (s)/(√(n))`

`=6.5\pm 0.9945\cdot (4)/(√(400))\\\\=6.5\pm 0.1989\\\\=(6.3011, 6.6989)\\\\\approx (6.3, 6.7)`

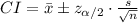
The 68% confidence interval is (6.3, 6.7).

The margin of error is:

`MOE=(UL-LL)/(2)=(6.7-6.3)/(2)=0.20`

(b)

Compute the 95% confidence interval for population mean as follows:

`CI=\bar x\pm z_(\alpha/2)\cdot (s)/(√(n))`

`=6.5\pm 1.96\cdot (4)/(√(400))\\\\=6.5\pm 0.392\\\\=(6.108, 6.892)\\\\\approx (6.1, 6.9)`

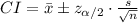
The 95% confidence interval is (6.1, 6.9).

The margin of error is:

`MOE=(UL-LL)/(2)=(6.9-6.1)/(2)=0.40`

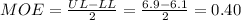
(c)

Compute the 99.7% confidence interval for population mean as follows:

`CI=\bar x\pm z_(\alpha/2)\cdot (s)/(√(n))`

`=6.5\pm 0.594\cdot (4)/(√(400))\\\\=6.5\pm 0.392\\\\=(5.906, 7.094)\\\\\approx (5.9, 7.1)`

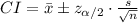
The 99.7% confidence interval is (5.9, 7.1).

The margin of error is:

`MOE=(UL-LL)/(2)=(7.1-5.9)/(2)=0.55`