Question

The graph of f(x) = x2 is shifted 3 units to the left to obtain the graph of g(x). Which of the following equations best describes g(x)?

g(x) = (x + 3)2
g(x) = x2 − 3
g(x) = x2 + 3
g(x) = (x − 3)2

Answer 1

g(x) = (x + 3)^2

Explanation:

I took the practice test as well.

Answer 2

g(x) = (x + 3)^2

Explanation:

When a function is shifted to the left or right we can think of a change of units, like a new independent variable is used.

If we have a quadratic function

`f(x)=x^2`

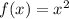
and the graph is shifted 3 units to the left, then we can think of a new unit u that is:

`g(x)=u^2`

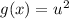
The vertix of this parabola, that happens for u=0, happens for x=-3, as the graph is shifted 3 units to the left.

Then, the relation between u and x is:

`u-x=0-(-3)=3\\\\u=x+3`

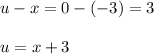
We can use this relation in the new graph and replace u as:

`g(x)=(x+3)^2`