Question

Fill out the following table. Do you see a pattern? Do you think this will always be true? Why or why not?

*Trolling answers will be flagged & reported, thank you.*

Answer 1

Please see table completed attached

Yes, there are several patterns, since these two trigonometric functions are periodic with same periodicity, and also satisfy the Pythagorean identity for the same angle.

Explanation:

Notice that we are asked about a table of trigonometric functions for the so called "special angles" which render values associated with half of the square root of a counting number between 0 and 4:

`(√(0) )/(2)=0 \\(√(1) )/(2)=(1)/(2) \\(√(2) )/(2)\\ (√(3) )/(2)\\ (√(4) )/(2)=(2)/(2) =1`

These two trigonometric functions also satisfy the Pythagorean identity for any angle
`\theta` in the unit circle, so the equality to one will always be true:

`sin^2(\theta)+cos^2(\theta)=1`

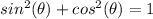
They are also periodic functions of period
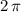`2\,\pi`, so their resulting values will be repeated with that periodicity.