Question

Carbon–14 is a radioactive isotope that decays exponentially at a rate of 0.0124 percent a year. How many years will it take for carbon–14 to decay to 10 percent of its original amount? The equation for exponential decay is At = A0e–rt.

Answer 1

It will take 18,569.2 years for carbon–14 to decay to 10 percent of its original amount

Explanation:

The amount of Carbon-14 after t years is given by the following equation:

`A(t) = A(0)e^(-rt)`

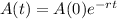
In which A(0) is the initial amount and r is the decay rate, as a decimal.

Carbon–14 is a radioactive isotope that decays exponentially at a rate of 0.0124 percent a year.

This means that
`r = (0.0124)/(100) = 0.000124`

How many years will it take for carbon–14 to decay to 10 percent of its original amount?

This is t for which:

`A(t) = 0.1A(0)`

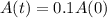
So

`A(t) = A(0)e^(-rt)`

`0.1A(0) = A(0)e^(-0.000124t)`

`e^(-0.000124t) = 0.1`

`\ln{e^(-0.000124t)} = ln(0.1)`

`-0.000124t = ln(0.1)`

`t = -(ln(0.1))/(0.000124)`

`t = 18569.2`

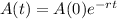
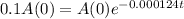
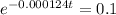
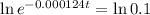
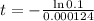
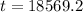
It will take 18,569.2 years for carbon–14 to decay to 10 percent of its original amount