Question

Sue has a crate, open at the top, in the shape of a cuboid. The internal dimensions of the crate are 36cm long by 36cm wide by 60cm high. Sue has a stick of length 90cm. She places the stick in the crate so that the shortest possible length extends out above the top of the crate. A) Calculate the length of the stick that extends out of the crate. B) Calculate the angle the stick makes with the base of the crate.

Answer 1

Answer: This is the answer to B) 47.9 degrees

Explanation:

Pythagoras: a^2+b^2=c^2

36²+36²=C²

√2592=C

C=50.9cm

Used Trigonometry: SOH CAH TOA

Tan=Opposite/Adjacent

Tan=60/50.9

The angle stick makes when it meets the base:

Tan^-1(60/50.9)

=49.7˚

Answer 2

Explanation:

When the stick is placed along the diagonal of the cuboid , shortest possible length will extend out above top of the crate .

Length of the diagonal

=
`√(36^2+36^2+60^2)`

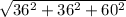
= 78.69 cm

the length of the stick that extends out of the crate

= 90 - 78.69

= 11.31 cm

If θ be the angle made by stick with the base

cosθ = hypotenuse of base / diagonal of cuboid

=
`(√(2)*36 )/(78.69)`

=
`(50.90 )/(78.69)`

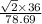
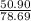
θ = 50°