Answer:
The solution of the system of equations is (x,y) = (2,0)
Explanation:
The equation of a line through the points
 and
and
 is equal to:
is equal to:

Where
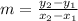
So, the equation of the line through the points (3, 1) and (–5, –7) is:
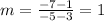
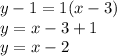
Then, we have two equations, y=x-2 and y=0.5x -1 , so solving for x, we get:
x - 2 = 0.5 x - 1
x - 0.5x = 2 - 1
x = 2
Replacing x=2 in the equation y=x-2, we get:
y =2 - 2 = 0
Finally, the solution of the system of equations is (x,y) = (2,0)