Question

Please assist me with the missing answers. Scores on the final exam in Ms. Meyer's statistics class follow a normal distribution, with a mean of 82 and a standard deviation of 5. 1. Ron, who took Ms Meyer's class, scored 88 on the final. Ron's z-score is + ___ 2. This means that Ron's final score is A. 1.2 points above the mean B. 1.2 standard deviation above the mean C. 1.2 points below the mean D 1.2 standard deviation below the mean

Answer 1

Answer: Ron's z-score is +1.2.

B. 1.2 standard deviation above the mean .

Explanation:

Let X deontes the scores of the final exam

Given : Ms. Meyer's statistics class follow a normal distribution with Mean = 82

standard deviation =5.1

Formula :
`z=(X-Mean)/(Standard \ deviation)`

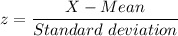
Ron scored 88 on the final.

So, put x=88

`z=(88-82)/(5.1)=(6)/(5.1)\approx1.2`

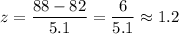
Ron's z-score is +1.2.

Also, 88= 86+(1.2 x 5.1) = Mean +1.2 x (standard deviation)

i.e. This means that Ron's final score is 1.2 standard deviation above the mean .