Question

Suppose a space vehicle with a rest mass of 150 000 kg travels past the International Space Station at a constant speed of 2.6 x 108 m/s with respect to the I.S.S. When an observer on the I.S.S. measures the moving vehicle, her measurement of the space vehicle length is 25.0 m. Determine the relativistic mass of the space vehicle. Determine the length of the space vehicle as measured by an astronaut on the space vehicle.

Answer 1

m = 300668.9 kg

L₀ = 12.47 m

Step-by-step explanation:

The relativistic mass of the space vehicle is given by the following formula:

`m = \frac{m_(0)}{\sqrt{1-(v^(2) )/(c^(2)) } }`

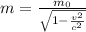
where,

m = relativistic mass = ?

m₀ = rest mass = 150000 kg

v = relative speed = 2.6 x 10⁸ m/s

c = speed of light = 3 x 10⁸ m/s

Therefore

`m = \frac{150000kg}{\sqrt{1-((2.6 x 10^(8)m/s)^(2) )/((3 x 10^(8)m/s)^(2)) } }`

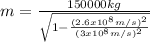
m = 300668.9 kg

Now, for rest length of vehicle:

L = L₀√(1 - v²/c²)

where,

L = Relative Length of Vehicle = 25 m

L₀ = Rest Length of Vehicle = ?

Therefore,

25 m = L₀√[1 - (2.6 x 10⁸ m/s)²/(3 x 10⁸ m/s)²]

L₀ = (25 m)(0.499)

L₀ = 12.47 m