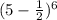Question

For the given term, find the binomial raised to the power, whose expansion it came from: 15(5)^2 (-1/2 x) ^4

A. (5+1/2 x)^6
B. (Y- 1/2 x) ^6
C. (5- 1/2 x) ^6
D. (-5 + (- 1/2 x))^6

Answer 1

C.
`(5-(1)/(2))^6`

Explanation:

Given

`15(5)^2(-(1)/(2))^4`

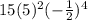
Required

Determine which binomial expansion it came from

The first step is to add the powers of he expression in brackets;

`Sum = 2 + 4`

`Sum = 6`

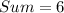
Each term of a binomial expansion are always of the form:

`(a+b)^n = ......+ ^nC_ra^(n-r)b^r+.......`

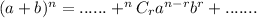
Where n = the sum above

`n = 6`

Compare
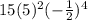`15(5)^2(-(1)/(2))^4` to the above general form of binomial expansion

`(a+b)^n = ......+15(5)^2(-(1)/(2))^4+.......`

Substitute 6 for n

`(a+b)^6 = ......+15(5)^2(-(1)/(2))^4+.......`

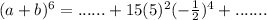
[Next is to solve for a and b]

From the above expression, the power of (5) is 2

Express 2 as 6 - 4

`(a+b)^6 = ......+15(5)^(6-4)(-(1)/(2))^4+.......`

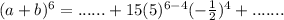
By direct comparison of

`(a+b)^n = ......+ ^nC_ra^(n-r)b^r+.......`

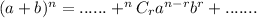
and

`(a+b)^6 = ......+15(5)^(6-4)(-(1)/(2))^4+.......`

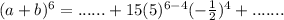
We have;

`^nC_ra^(n-r)b^r= 15(5)^(6-4)(-(1)/(2))^4`

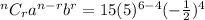
Further comparison gives

`^nC_r = 15`

`a^(n-r) =(5)^(6-4)`

`b^r= (-(1)/(2))^4`

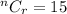
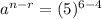
[Solving for a]

By direct comparison of
`a^(n-r) =(5)^(6-4)`

`a = 5`

`n = 6`

`r = 4`

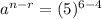
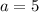
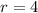
[Solving for b]

By direct comparison of
`b^r= (-(1)/(2))^4`

`r = 4`

`b = (-1)/(2)`

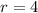
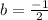
Substitute values for a, b, n and r in

`(a+b)^n = ......+ ^nC_ra^(n-r)b^r+.......`

`(5+(-1)/(2))^6 = ......+ ^6C_4(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+ ^6C_4(5)^(6-4)((-1)/(2))^4+.......`

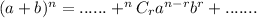
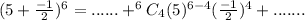
Solve for
`^6C_4`

`(5-(1)/(2))^6 = ......+ (6!)/((6-4)!4!))*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+ (6!)/(2!!4!)*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+ (6*5*4!)/(2*1*!4!)*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+ (6*5)/(2*1)*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+ (30)/(2)*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+15*(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+15(5)^(6-4)((-1)/(2))^4+.......`

`(5-(1)/(2))^6 = ......+15(5)^2((-1)/(2))^4+.......`

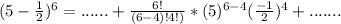
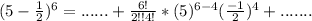
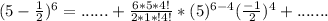
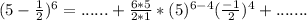
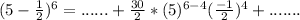
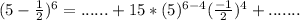
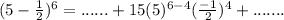
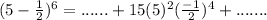
Check the list of options for the expression on the left hand side

The correct answer is
`(5-(1)/(2))^6`