Question

An astronaut is in equilibrium when he is positioned 140 km from the center of asteroid X and 481 km from the center of asteroid Y, along the straight line joining the centers of the asteroids. What is the ratio of the masses X/Y of the asteroids

Answer 1

Step-by-step explanation:

It is given that, An astronaut is in equilibrium when he is positioned 140 km from the center of asteroid X and 481 km from the center of asteroid Y, along the straight line joining the centers of the asteroids. We need to find the ratio of their masses.

As they are in equilibrium, the force of gravity due to each other is same. So,

`(Gm_xM)/(r^2)=(Gm_yM)/(r^2)\\\\(m_x)/(r_x^2)=(m_y)/(r_y^2)\\\\(m_x)/(r_x^2)=(m_y)/(r_y^2)\\\\(m_x)/(m_y)=((r_x^2)/(r_y^2))\\\\(m_x)/(m_y)=((140^2)/(481^2))\\\\(m_x)/(m_y)=0.0847`

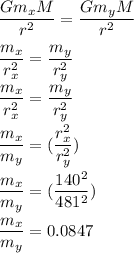
So, the ratio of masses X/Y is 0.0847