Answer:
Wavelength is 0.359 m
Step-by-step explanation:
Given that,
Magnetic field, B = 0.547 T
We need to find the wavelength of radiation produced by a proton in a cyclotron with a magnetic field of 0.547 T.
The frequency of revolution of proton in the cyclotron is given by :

m is mass of proton
q is charge on proton
So,
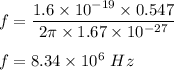
We know that,
Speed of light,
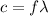
 = wavelength
= wavelength
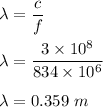
So, the wavelength of the radiation produced by a proton is 0.359 m.