Question

A box with a hinged lid is to be made out of a rectangular piece of cardboard that measures 3 centimeters by 5 centimeters. Six squares will be cut from the cardboard: one square will be cut from each of the corners, and one square will be cut from the middle of each of the -5 centimeter sides . The remaining cardboard will be folded to form the box and its lid . Letting x represent the side-lengths (in centimeters) of the squares, to find the value of that maximizes the volume enclosed by this box. Then give the maximum volume. Round your responses to two decimal places.

Answer 1

The volume of the box is maximized when x = 0.53 cm

Therefore, x = 0.53 cm and the Maximum volume = 1.75 cm³

Step-by-step explanation:

Please refer to the attached diagram:

The volume of the box is given by

`V = Length * Width * Height \\\\`

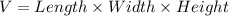
Let x denotes the length of the sides of the square as shown in the diagram.

The width of shaded region is given by

`Width = 3 - 2x \\\\`

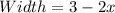
The length of shaded region is given by

`Length = (1)/(2) (5 - 3x) \\\\`

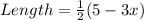
So, the volume of the box becomes,

`V = (1)/(2) (5 - 3x) * (3 - 2x) * x \\\\V = (1)/(2) (5 - 3x) * (3x - 2x^2) \\\\V = (1)/(2) (15x -10x^2 -9 x^2 + 6 x^3) \\\\V = (1)/(2) (6x^3 -19x^2 + 15x) \\\\`

Take the derivative of volume and set it to zero.

`(dV)/(dx) = 0 \\\\(dV)/(dx) = (d)/(dx) ( (1)/(2) (6x^3 -19x^2 + 15x)) \\\\(dV)/(dx) = (1)/(2) (18x^2 -38x + 15) \\\\(dV)/(dx) = (1)/(2) (18x^2 -38x + 15) \\\\0 = (1)/(2) (18x^2 -38x + 15)\\\\18x^2 -38x + 15 = 0\\\\`

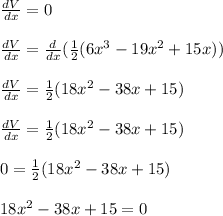
We may solve the quadratic equation using the quadratic formula.

`$x=(-b\pm√(b^2-4ac))/(2a)$`

The values of coefficients a, b, c are

`a = 18 \\\\b = -38 \\\\c = 15 \\\\`

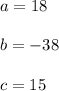
Substituting the values into quadratic formula yields,

`x=(-(-38)\pm√((-38)^2-4(18)(15)))/(2(18)) \\\\x=(38\pm√((1444- 1080))/(36) \\\\x=(38\pm√((364))/(36) \\\\x=(38\pm 19.078)/(36) \\\\x=(38 + 19.078)/(36) \: or \: x=(38 - 19.078)/(36)\\\\x= 1.59 \: or \: x = 0.53 \\\\`

Volume of box when x = 1.59:

`V = (1)/(2) (5 - 3(1.59)) * (3 - 2(1.59)) * (1.59) \\\\V = -0.03 \: cm^3 \\\\`

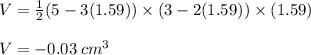
Volume of box when x = 0.53:

`V = (1)/(2) (5 - 3(0.53)) * (3 - 2(0.53)) * (0.53) \\\\V = 1.75 \: cm^3`

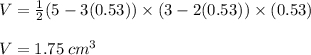
As you can see, the volume of the box is maximized when x = 0.53 cm

Therefore, x = 0.53 cm and the Maximum volume = 1.75 cm³