Answer:
The ratio of the average change in profit when the level of production changes from 600 to 620 units per month is -24 : 5.
Explanation:
The question is:
A company knows that if it produces "x" monthly units its utility "u" could be calculated with the expression: u (x) = - 0.04x ^ 2 + 44x-4000 where "u" is expressed in dollars. Determine the ratio of the average change in profit when the level of production changes from 600 to 620 units per month. Remember that the slope of the secant line to the graph of the function represents the average rate of change.
Solution:
The expression for the utility is:
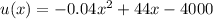
It is provided that the slope of the secant line to the graph of the function represents the average rate of change.
Then the ratio of the average change in profit when the level of production changes is:

Compute the values of u (x₁) and u (x₂) as follows:
x₁ = 600
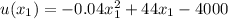
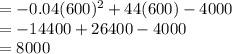
x₂ = 620


Compute the average rate of change as follows:

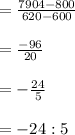
Thus, the ratio of the average change in profit when the level of production changes from 600 to 620 units per month is -24 : 5.