Answer:
The perimeter of the figure is: 8x + 34.
Explanation:
The perimeter of a shape is the sum of the length of all its sides. In this case there is one side missing, we need to find its length. To do that we will have to use Pythagora's theorem, because we will create a right triangle as shown in the attached picture. Where a, b and c are the sides of the right triangle. We can determine the lengths of a and c. If we pay close attention to the figure we will realize that a is:
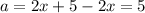
While c is:
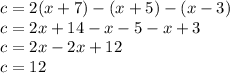
We can now apply Pythagora's theorem:
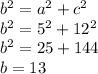
With this we can sum all the sides and calculate the perimeter of the shape.
