Question

Write an equation in slope-intercept form of the line that passes through the point (-6,-5) with slope 6.

Answer 1

y=6x+31

Explanation:

Since we are given a point and a slope, we can use the slope-intercept formula.

`y-y_(1) =m(x-x_(1))`

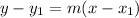
where (x1,y1) is a point on the line and m is the slope.

The point given is (-6,-5) and the slope is 6.

x1= -6

y1= -5

m=6

`y--5=6(x--6)`

A negative number subtracted from another number, or two negative signs, becomes a positive.

`y+5=6(x+6)`

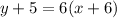
We want to find the equation of the line, which is y=mx+b (m is the slope and b is the y-intercept). Therefore, we must get y by itself on one side of the equation.

First, distribute the 6. Multiply each term inside the parentheses by 6.

`y+5=(6*x)+(6*6)`

`y+5=6x+36`

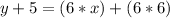
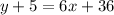
Subtract 5 from both sides, because it is being added on to y.

`y+5-5=6x+36-5`

`y=6x+36-5`

`y=6x+31`

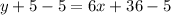
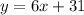
The equation of the line is y=6x+31