Question

Please help thank you

Answer 1

a = 4

b = 11

Explanation:

To convert the recurring decimals into fraction we will follow the following process.

Given recurring decimal is 0.36363636....

Let x = 0.363636......... (1)

Multiply this decimal with 100.

100x = 36.36363636...........(2)

Now subtract expression (1) from expression (2)

100x = 36.363636.......

x = 0.363636.......

99x = 36

x =
`(36)/(99)`

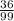
x =
`(4)/(11)`

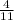
Since,
`x=(a)/(b)=(4)/(11)`

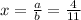
a = 4 and b = 11 will be the answer.