Answer:
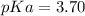
Step-by-step explanation:
Hello,
In this case, given the information, we can compute the concentration of hydronium given the pH:
![pH=-log([H^+])\\](https://img.qammunity.org/2021/formulas/chemistry/college/av02t5rd5ylg8m6mzai95k32ef3v96wtlu.png)
![[H^+]=10^(-pH)=10^(-2.18)=6.61x10^(-3)M](https://img.qammunity.org/2021/formulas/chemistry/college/1dq1gs9l2ydn1a9kwp8nhpo4rn5rl52hiy.png)
Next, given the concentration of the acid and due to the fact it is monoprotic, its dissociation should be:

We can write the law of mass action for equilibrium:
![Ka=([H^+][A^-])/([HA])](https://img.qammunity.org/2021/formulas/chemistry/college/kzt7pcnoonz2fwfrgadywivsijtflo2dmk.png)
Thus, due to the stoichiometry, the concentration of hydronium and A⁻ are the same at equilibrium and the concentration of acid is:
![[HA]=0.2230M-6.61x10^(-3)M=0.2164M](https://img.qammunity.org/2021/formulas/chemistry/college/eaej8r8ps2rf60m16dxbwemarl4sloagni.png)
As the concentration of hydronium also equals the reaction extent (
 ). Thereby, the acid dissociation constant turns out:
). Thereby, the acid dissociation constant turns out:
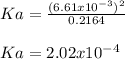
And the pKa:

Regards.